If v(x,y))=(1-2xy+y^2)^(-1/2) and x ∂v/∂x-y ∂v/∂y=y^2 v^k,then find k

If v(x,y))=(1-2xy+y^2)^(-1/2) and x del v/del x-y del v/del y=y^2 v^k, then find k.
If v(x,y))=(1-2xy+y^2)^(-1/2) and x ∂v/∂x-y ∂v/∂y=y^2 v^k,then find k.

Question

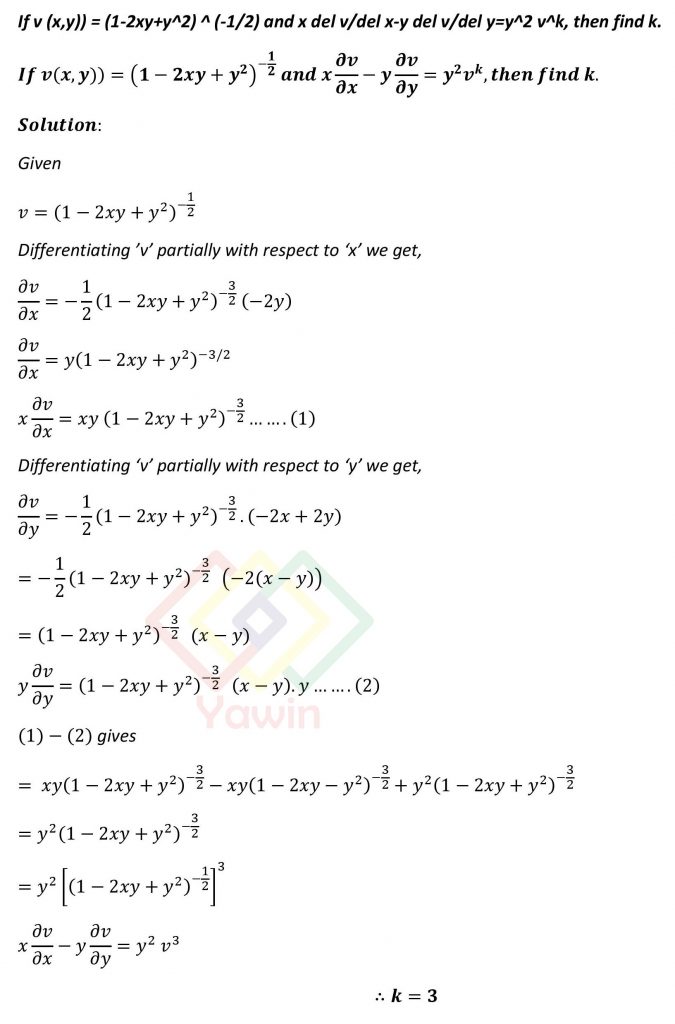
Question

If v(x,y))=(1-2xy+y^2)^(-1/2) and x del v/del x-y del v/del y=y^2 v^k, then find k.
If v(x,y))=(1-2xy+y^2)^(-1/2) and x ∂v/∂x-y ∂v/∂y=y^2 v^k,then find k.

Euler’s theorem Problems

1. If u=(x^3+y^3)/sqrt(x+y) prove that x du/dx + y du/dy = 5/2 uIf u=(x^3+y^3)/sqrt(x+y) prove that x del u/del x + y del u/del y = 5/2 uprove that  x ∂u/∂x+y ∂u/∂y=5/2 u
2. If u=√(x^4+y^4) tan^-1(y/x), Show that x u_x+y u_y=2u=x^2 u_xx+2xy u_xy+y^2 u_yy
   If u=√(x^4+y^4) tan^(-1)⁡(y/x) Show that xu_x+yu_y=2u=x^2 u_xx+2xy uxy+y^2 u_yy
3. If u=log((x^4+y^4)/(x+y)) Show that x del u/ del x+y del u/ del y = 3
   If u=log((x^4+y^4)/(x+y)), Show that x ∂u/∂x+y ∂u/∂y=3 using Euler’s theorem
4. If u=tan^(-1)((x^3+y^3)/(x+y)),
   Show that
   1. x u_x+y u_y = sin2u
   2. x^2 u_xx+2x_y u_xy+y^2 u_yy=sin4u-sin2uIf u=tan^(-1)⁡((x^3+y^3)/(x+y)),
   Show that
   1.xu_x+yu_y=sin2u
   2.x^2 u_xx+2x_y u_xy+y^2 u_yy=sin4u-sin2u
5. if u=sin^(-1)((x^2+y^2)/(x+y)), Show that x del u/del x+y del u/del y=tan u
   If u=sin^(-1)⁡((x^2+y^2)/(x+y)), Show that x ∂u/∂x+y ∂u/∂y=tan u
6. If u=cosec^(-1) ((x^(1/2)+y^(1/2))/(x^(1/3) +y^(1/3) )), Show that x.del u/del x+y.del u/del y=(-1)/6.tan uIf u=cosec^(-1) ((x^(1/2)+y^(1/2))/(x^(1/3)+y^(1/3) )),Show that x.∂u/∂x+y.∂u/∂y=(-1)/6 tan⁡u
7. State Euler’s theorem and use it to find, x del u/del x+y del u/del y when u=tan^(-1) ((x^2+y^2)/(x+y))State Euler’s theorem and use it to find, x∂u/∂x+y∂u/∂y when u=tan^(-1)((x^2+y^2)/(x+y))
8. If u=sin^(-1)((x+2y+3z)/(x^8+y^8+z^8)) then find x del u/del x+y del u/del y+z del u/del z
   If u=sin^(-1)((x+2y+3z)/(x^8+y^8+z^8)) then find x ∂u/∂x+y ∂u/∂y+z ∂u/∂z
9. If v(x,y))=(1-2xy+y^2)^(-1/2) and x del v/del x-y del v/del y=y^2 v^k, then find k.
   If v(x,y))=(1-2xy+y^2)^(-1/2) and x ∂v/∂x-y ∂v/∂y=y^2 v^k,then find k.