Change the order of integration and hence evaluate integral of dx dy over the limits x=(sqrt(y) to 1) and y=(0 to 1)
Question
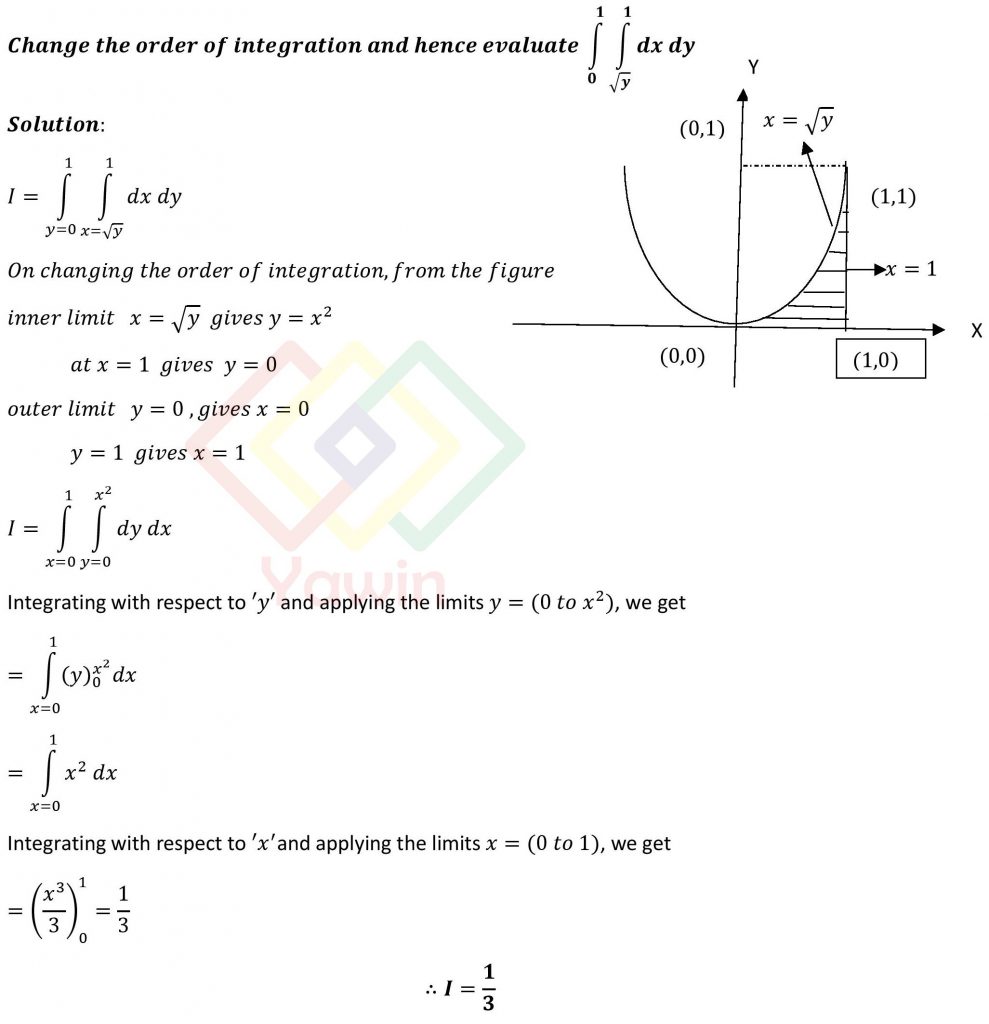
Question
Change the order of integration and hence evaluate integral of dx dy over the limits x=(sqrt(y) to 1) and y=(0 to 1)
Topic
Types of Evaluation of Double Integral
Problems
- Evaluate double integral of xy dx dy over the specified region R, where R is the region bounded by the coordinate axes and the line x+y=1
- Evaluate double integral of [xy(x+y) dy dx ] taken over the area between y=x^2 and y=x
- Evaluate double integral of xy dx dy over the positive quadrant of the circle x^2+y^2=a^2
- Evaluate double integral of xy dy dx over the limits x=(0 to 1) and y=(x to sqrt(x)) by changing the order of integration
- Change the order of integration and hence evaluate integral of dx dy over the limits x=(sqrt(y) to 1) and y=(0 to 1)
- Change the order of integration and hence evaluate double integral of xy dy dx over the limits y= (x^2/4a to 2xsqrt(ax)) and x = (o to 4a)
- Evaluate double integral of e^(x^2+y^2) over the limits x=(0 to infinity) and y=(0 to infinity) by changing into polar coordinates
- Evaluate e^-y / y dy dx over the limits y = (x to infinity) and x = (0 to infinity) by changing order of integration
- Evaluate x/(x^2+y^2) over the limits x=(y to a) and y=(0 to a) by changing the order of integration
- Change the integral sqrt (x^2+y^2) over the limits y=(0 to sqrt(a^2-x^2) ans x=(-a to a) into polars and hence evaluate its value
